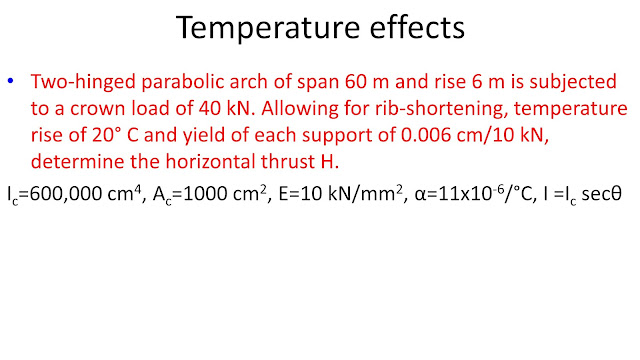
Flexibility Matrix Method

 FLEXIBILITY MATRIX METHOD

This is matrix implementation of force method of analysis. The procedure assumes that the structural system is linear elastic. Because of this assumption, the application of principle of superposition is valid.

For a system having a single coordinate (Known Force)

System Flexibility Matrix Generation

Flexibility coefficient f_ij is defined as the displacement (can be deflection or rotation or relative rotation or relative translation) along coordinate i, due to unit force (can be force or moment or bending moment or shear) along coordinate j. 

Thus, to determine any flexibility coefficient we need to evaluate the displacements in various locations and directions. For this purpose, we can use double integration method,  moment area method or conjugate beam method, unit load method or strain energy method. For one or two span beams, application of conjugate beam is convenient. But, in general, strain energy or unit load method is more applicable. 

Illustrative Example#1

Q: Derive the flexibility matrix for the beam shown below 

INtroduction to Dynamics of Structures

 WELCOME 

to 

Dynamics of Structures

Contents

• Syllabus- Handouts-
• Unit-I
• Unit-II
• Unit-III
• Unit-IV
• Unit-V

Introduction to THeory of Plates and shells

 WELCOME 

to 

The theory of Plates and Shells

• Syllabus-
• Unit- I
• Uni

Analysis of Indeterminate Arches

 Review: Analysis of thee-hinged arches of various shapes is given here.

Outline of the blog post

• Degree of static Indeterminacy in arches
• Use of force method to solve for redundant reaction (Especially Horizontal Thrust H)
• For two hinged arches
• For symmetric arches without Horizontal load
• For parabolic arches with secant variation of MI
• For single concentrated load at c distance
• For any other loading using the result of single concentrated load
• For segmental arches
• For fixed arches
• For temperature effects