Analysis of determinate arches for external and internal reactions

Introduction to arches : Click here to view the blog post on introduction to arches

In this blog post, we will see problems illustrating the principles of analysis of determinate arches. In this discussion, Analysis of arches means analysing an arch for evaluating the force effects of loading (i.e reactions at supports, internal reactions like axial force or thrust, transverse shear or radial shear  and bending moments at various sections of the arch

Before starting the example, let us see the formulations for common shapes of arches

Parabolic arch: In the figure below, you can see the formula describing the parabolic arch shape. Please NOTE that the origin is at A. That means you are measuring all the horizontal and vertical distances from A.

Circular or segmental arch: The figure below shows the formulation for segmental arch. NOTE that it is sometimes easy to use the polar coordinates instead of cartesian coordinates. You can see in the inset of the figure, a very useful property of chord of a circle.

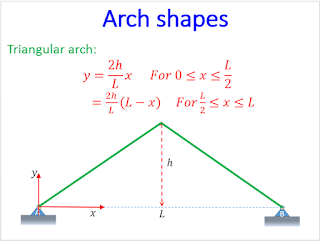
Triangular Arch: In the figure below, you can see the formulation for triangular arch. NOTE that the expression will be different for the left and right portions. 

Let us start the example with a simple symmetrical parabolic three-hinged arch, as shown below

The first step in the solution is to find reactions using equilibrium equations. As discussed in previous blog post, we need to apply equilibrium equation 1) on the whole arch and 2) on the portion of arch separated at the internal hinge. The first part of this step is shown below

Horizontal force equilibrium is shown below

Moment equilibrium is applied as shown below

 NOTE that when the supports are at the same level, the horizontal reaction at B does not cause a moment at A.

Now, we need to apply equilibrium on the portion CB, separated at the location of internal hinge

NOTE: It is easy to always follow the strategy of taking moment about A for whole arch ACB and taking moment about the internal hinge C for the portion CB. By doing like this we get two equations in terms of V_B and H_B. Other options will make the analysis more lengthy and cumbersome.

Now we got all the reactions and we can solve for internal forces. We only need to draw BMD. So, we need to get expression for M_x only. since there is one concentrated load, we need to cut a section on the left of the load and one section on the right side of the load as shown below. By applying moment equilibrium for each of these sections, we get two expressions for M_x .

For the above section, it is easy to apply equilibrium for the portion on the left of the section, since this FBD has only two forces whose moments contribute to M_x . If we consider the equilibrium of portion XB on the right side of the section, we need to take moments of three forces to get M_x.

 Note that for the section on the right side of the load W, it is easy to consider the equilibrium of the portion on the right side of the section.

For both the sections, expression for M_x has two terms. We can draw the BMD by superimposing the plots of the two terms, as shown below

NOTE that in the above figure, the blue colour diagram (triangle) represents the first term (i.e 60x  and 20(20-x)  ) of the expressions for M_x.The red color parabola describes the second term of the expressions (i.e., 40y). We need to substract the two terms. So, the overlapping part is to be cancelled. The net BMD consists of the blue portion (positive) and the red portion (negative)

NOTE: THe maximum positive BM occurs under the concentrated load (in this case). Its value needs to be evaluated and shown on the net BMD diagram.

This section presents more examples of determinate arch analysis

EXAMPLE 1: UNSYMMETRICAL SEGMENTAL ARCH

 This is a segmental arch (an arch which is a part of a circle). Since the supports are at different heights, it is referred to as unsymmetrical arch.

The first step is to find the reactions, using 1) FBD of whole arch ACB and 2) FBD of portion CB

 NOTE; Unlike in arches with supports at same level, the moment about support A involves the moment caused by horizontal reaction (thrust) H_B . Especially for such problems as this, it will be easier if we  take moment equilibrium for whole arch ACB and portion CB, such that both equations are in terms of two reactions (Here V_B and H_B). Alternate ways will make the problem lengthy.

Now consider the moment equilibrium of portion CB of the arch

Note that the concentrated load at C will not make any difference in the equilibrium of portion CB

Note that the above solution became easier since both the equilibrium equations are in terms of V_B and H_B only, without involving V_A

Now, we can determine the other reactions using the horizontal force equilibrium and vertical force equilibrium on the FBD of the whole arch shown previously

To determine the internal forces at a section, we need to cut a section at the required location and apply equilibrium equations on the FBD of the cut portion. Internal forces are first determined in terms of horizontal and vertical forces and then they are resolved in the tangential and normal directions to get normal thrust and radial shear respectively.

For transforming horizontal and vertical forces into tangential and radial directions, we need to know the angle made by the tangent with horizontal. If this angle is not given, we need to use the differentiation of the equation of the arch shape. In the present problem the angle made by the tangent is already given.

Note: The above transformation relationships between the internal forces is derived for the positive angle and so can be used for any part of the arch

EXAMPLE 2: UNSYMMETRICAL PARABOLIC ARCH

The solution for this problem starts with sketching the figure showing all the details given in the question, as shown below

Now, consider the equilibrium of the whole arch, to evaluate the reactions

The above step is the only difficult part of the solution

For transforming horizontal and vertical forces into tangential and radial directions, we need to know the angle made by the tangent with horizontal. If this angle is not given, we need to use the differentiation of the equation of the arch shape. This is left for you as assignment. after determining the angle the calculations should continue as shown below

That's it. the solution is completed. For drawing BMD of the arch we can add the BMD caused by vertical forces and the BMD caused by horizontal forces.