In this post, I explained the analysis of continuous beam. Continuous beams are indeterminate beams, and their analysis (using force method) requires use of deformation conditions (known as compatibility conditions). In the following video the derivation of the Clapeyron's theorem of Three Moments is explained This follows the general procedure of force methods
The application of clapeyron's theorem of three moments is illustrated using two examples in the video below.
IN the write-up that follows, the analysis of a continuous beam with fixed end supports is discussed. If the end support is a fixed support, it needs to be replaced with a fictitious span of infinite moment of inertia. This procedure is illustrated as follows
IN the above problem, the support A is fixed. So, that support is replaced with a fictitious span A'A. The fictitious span (A'A) will not have any load, and its span is not needed;although it can be assumed to be of finite value
In the above figure, the BMD of each span is also shown. Note that, in this procedure, each span is assumed to be simply supported. Since, Clapeyron's Theorem of Three Moments (TTM) is applied for two-spans at a time, we will apply TTM for spans 1 & 2, then for spans 2 & 3.
Note that M1 is not needed. M2 is equal to fixed end moment at A, MA, and M2 is equal to MB . Now we can apply TTM to span A'A&AB as follows
Then TTM can be applied to next two spans, AB-BC
Solving equations 1&2 simultaneously,
After the support moments are obtained, the vertical reactions can be determined by simple application of equilibrium equations.
BMD of the continuous beam can be drawn by superimposing the simply supported BMDs caused by given loading and the simply supported BMD caused by end moments
The theorem of three moments can also be applied to propped cantilever beams and fixed beams as illsutrated below




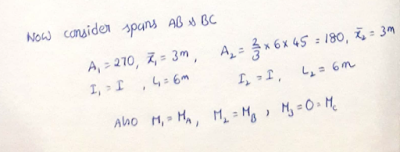




No comments:
Post a Comment